A decade-ish ago, I wrote an article entitled “The Nine Forms of the Five Room Dungeon”. The premise was simple: using specific rules of construction, there are only nine topologies to which a five room dungeon can conform. Ie: there are only nine five room dungeons (IF you follow those rules). Those rules are:
- Start with a room, the entrance to your dungeon.
- Choose an existing room. Add a hallway that leads to a new room. (Think of a lolipop)
- Repeat until you have five rooms.
These rules net us the following nine forms:
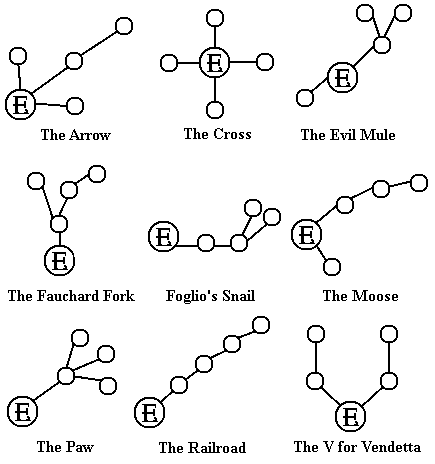
Readers were quick to point out that if you also abstracted the entrance, you actually only have Three forms: The Railroad, The Cross, and The Arrow.
Fast forward a decade, and reader Delsig left a comment pointing out the following: There is an academic paper on node topography that shows that without my simple rules of construction there are not three forms of the five room dungeon, nor nine, but still only twenty one.
You can read the paper here. I don’t pretend to understand the math, but the takeaway for us, is page two: the twenty one forms:
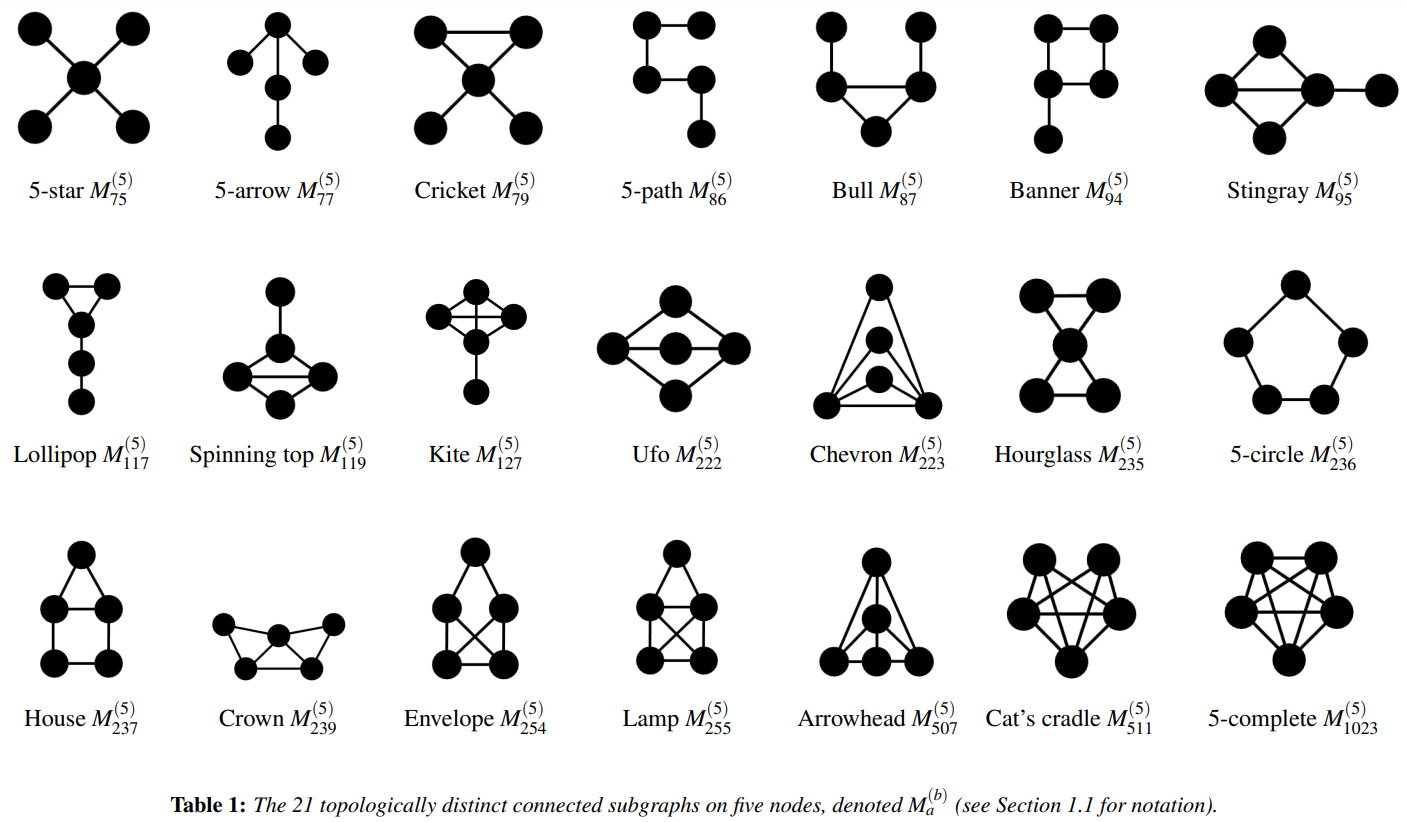
You can see the three forms from my original article in these twenty one as the 5 star, the 5 arrow, and the 5 path.
As a bonus, the paper also provides the two forms of the three room dungeon and the six forms of the four room dungeon (the one form of the one room dungeon and the one form of the two room dungeon are trivial).
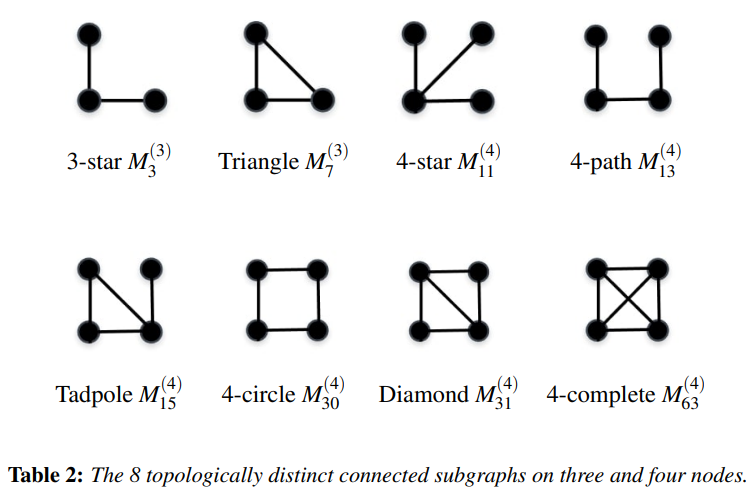
So if you want a small dungeon, choose one of these forms and that’s it. There aren’t any other forms that are topologically distinct from these. Choosing one is even fairly simple. The 21 forms lend themselves to a D20 roll (just discard one you don’t like) or if you add in the 2, 3, and 4 room options, you have a D30 roll all good to go.
Knowing there are only twenty one topologies is a real time saver, but the problem with the revelation that there are only twenty one forms the five room dungeon can take remains the same as it was a decade ago: twenty one forms is better than nine, but it collapses what seemed like an infinite variety into a rather small number of options. How do we keep our five room dungeons distinct now that we know there are really only twenty one possible topologies?
Just like last time, the devil is in the details:
- Rooms can be shifted around without changing the topography. Using the simplest example of the 5-path, the chart of the 21 forms has it in a question mark shape, but it could just as easily be a zig-zag or a straight line, or a spiral or…
- If you make the “hallways” stairs, the straight line is a wizard’s tower. Any or all of the connections in your dungeon could be elevation changes.
- Of course, there’s nothing saying these have to be dungeons. You can use these topographies for any purpose: ships, buildings, and so forth, as well as nonphysical representations like relationship maps, investigation trees, etc.
- The 6th room: Adding a 6th room and its attendant connections increases the number of topographies well beyond 21.
- Using 5 room segments as building blocks for larger structures can net you anything from a still small 10 room dungeon up to massive apartment buildings (I’ve lived in apartments with five rooms before), or megadungeons or similar.
Steve Lawford. Counting five-node subgraphs. 2021. ffhal-03097484f
















